Thermodynamic Expression of Economics
Expressing Cost, Revenue and Profit Thermodynamically
By Mark Ciotola
First published on May 17, 2019. Last updated on May 12, 2021.
Let’s start thinking about a heat engine and work in economic terms. Let’s imagine a firm or venture is analogous to a heat engine operating across a hot and a cold reservoir. Thermal energy removed from the hot reservoir \(Q_H\) is analogous to revenue (R). Thermal energy exhausted into the cold reservoir \(Q_C\) is analogous to cost (C). Work is analogous to profit. This analogy is shown below.
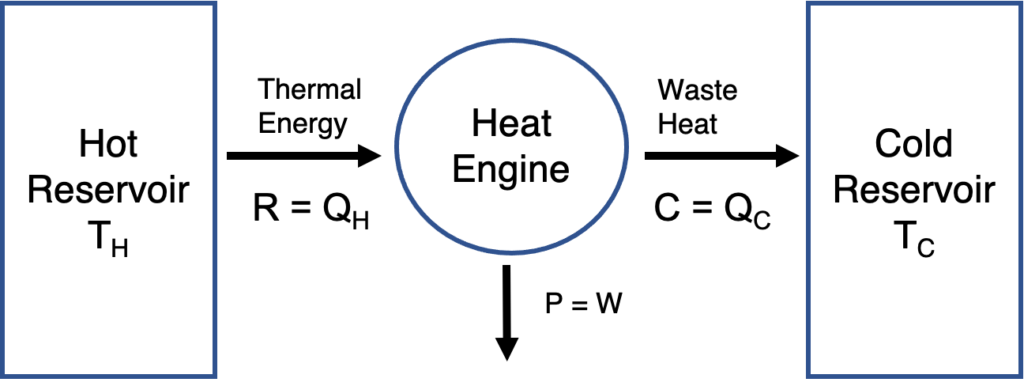
A venture modeled as a heat engine
Profits As Work
For example, you buy and sell widgets. You receive cash (revenue) when you sell widgets and you pay cash (costs) when you sell widgets. Here, there are no overhead costs. It’s a simple business.
Then, Profit P would be:
\(W = Q_H – Q_C\), or,
\(P = Q_H – Q_C\), or,
\(P = R – C = Q_H – Q_c\).
Gross Margin
Let’s make our analogy more realistic and interesting. Continuing to model our firm a heat engine operating across a thermal difference, let’s examine gross profit margins. In business, the Gross Profit Margin, or simply Gross Margin (MG), can be expressed as:
\(GM = \frac{R – C}{R}\)
The form of this equation may look familiar. It is identical in form to the expression for Carnot efficiency:
\(\epsilon = \frac{T_H – T_C}{T_C} =1 – \frac{T_C}{T_H}\)
So we can now express Gross Margin thermodynamically:
\(GM = \epsilon = 1 – \frac{T_C}{T_H}\)
We can now express Marginal Profit thermodynamically:
\(P =Q_H~\epsilon = Q_H~(1 -\frac{T_C}{T_H})\)
In Summary, the work performed by the heat engine represents profit (P):
\(W = Q_H (1 – \frac{T_C}{ T_H})\)
Or combining expressions:
In Summary, the work performed by the heat engine represents profit (P):
\(GM = W =R (1 – \frac{C}{ R}) = Q_H (1 – \frac{T_C}{ T_H})\)
« Costs, Revenues and Profits | COURSE | Marginal Revenue, Cost and Profit »